Reset password New user? Sign up
Existing user? Log in

Sets - Problem Solving
Already have an account? Log in here.
This wiki is incomplete.
If \(A = \{ 1,2,3,4 \}, B = \{ 4,5,6,7 \},\) determine the following sets: (i) \(A \cap B\) (ii) \(A \cup B\) (iii) \(A \backslash B \) (i) By definition, \(\cap\) tells us that we want to find the common elements between the two sets. In this case, it is 4 only. Thus \(A \cap B = \{ 4 \} \). (ii) By definition, \(\cup\) tells us that we want to combine all the elements between the two sets. In this case, it is \(A\cup B = \{1,2,3,4,5,6,7 \} \). (iii) By definition, \( \backslash \) tells us that we want to look for elements in the former set in that doesn't appear in the latter set. So \(A \backslash B = \{1,2,3\} \). \(_\square\)
Consider the same example above. If the element \(4\) is removed from the set \(B\), solve for (i), (ii), (iii) as well. (i) Since there is no common elements in sets \(A\) and \(B\), then \(A \cap B = \phi \) or \(A \cap B = \{ \} \). (ii) Because the element \(4\) is no longer repeated, then \(A \cup B \) remains the same. (iii) Since \(A\) and \(B\) no longer share any common element, \(A\backslash B \) is simply equals to set \(A\), which is \(\{1,2,3,4 \} \). \(_\square\)
If \(P=\{2, 5, 6, 3, 7\}\) and \(Q=\{1, 2, 3, 8, 9, 10\},\) which of the following Venn diagrams represents the relationship between the two sets?
\[\large\color{darkred}{B=\{ \{ M,A,T,H,S \} \}}\]
Find the cardinal number of the set \(\color{darkred}{B}\).
Note: The cardinal number of a set is equal to the number of elements contained in the set.
Bonus question given with the picture.
Join the brilliant classes and enjoy the excellence. also checkout foundation assignment #2 for jee..
Consider the set \( \lbrace{1, 2, 3, 4, 5, 6, 7, 8, 9, 10\rbrace}\).
For each of its subsets, let \( M \) be the greatest number. Find the last three digits of the sum of all the \( M \)'s.
Assume that \(0\) is the greatest number of the empty subset.
The number of subsets in set A is 192 more than the number of subsets in set B. How many elements are there in set A?
Problem Loading...
Note Loading...
Set Loading...
WORD PROBLEMS ON SETS AND VENN DIAGRAMS
Basic stuff.
To understand, how to solve Venn diagram word problems with 3 circles, we have to know the following basic stuff.
u ----> union (or)
n ----> intersection (and)
Addition Theorem on Sets
Theorem 1 :
n(AuB) = n(A) + n(B) - n(AnB)
Theorem 2 :
=n(A) + n(B) + n(C) - n(AnB) - n(BnC) - n(AnC) + n(AnBnC)
Explanation :
Let us come to know about the following terms in details.
n(AuB) = Total number of elements related to any of the two events A & B.
n(AuBuC) = Total number of elements related to any of the three events A, B & C.
n(A) = Total number of elements related to A
n(B) = Total number of elements related to B
n(C) = Total number of elements related to C
For three events A, B & C, we have
n(A) - [n(AnB) + n(AnC) - n(AnBnC)] :
Total number of elements related to A only
n(B) - [n(AnB) + n(BnC) - n(AnBnC)] :
Total number of elements related to B only
n(C) - [n(BnC) + n(AnC) + n(AnBnC)] :
Total number of elements related to C only
Total number of elements related to both A & B
n(AnB) - n(AnBnC) :
Total number of elements related to both (A & B) only
Total number of elements related to both B & C
n(BnC) - n(AnBnC) :
Total number of elements related to both (B & C) only
Total number of elements related to both A & C
n(AnC) - n(AnBnC) :
Total number of elements related to both (A & C) only
For two events A & B, we have
n(A) - n(AnB) :
n(B) - n(AnB) :

Solved Problems
Problem 1 :
In a survey of university students, 64 had taken mathematics course, 94 had taken chemistry course, 58 had taken physics course, 28 had taken mathematics and physics, 26 had taken mathematics and chemistry, 22 had taken chemistry and physics course, and 14 had taken all the three courses. Find how many had taken one course only.
Let M, C, P represent sets of students who had taken mathematics, chemistry and physics respectively.
From the given information, we have
n(M) = 64, n(C) = 94, n(P) = 58,
n(MnP) = 28, n(MnC) = 26, n(CnP) = 22
n(MnCnP) = 14
From the basic stuff, we have
Number of students who had taken only Math
= n(M) - [n(MnP) + n(MnC) - n(MnCnP)]
= 64 - [28 + 26 - 14]
Number of students who had taken only Chemistry :
= n(C) - [n(MnC) + n(CnP) - n(MnCnP)]
= 94 - [26+22-14]
Number of students who had taken only Physics :
= n(P) - [n(MnP) + n(CnP) - n(MnCnP)]
= 58 - [28 + 22 - 14]
Total n umber of students who had taken only one course :
= 24 + 60 + 22
Hence, the total number of students who had taken only one course is 106.
Alternative Method (Using venn diagram) :
Venn diagram related to the information given in the question:
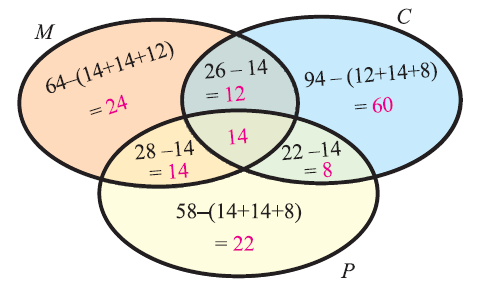
From the venn diagram above, we have
Number of students who had taken only math = 24
Number of students who had taken only chemistry = 60
Number of students who had taken only physics = 22
Total Number of students who had taken only one course :
Problem 2 :
In a group of students, 65 play foot ball, 45 play hockey, 42 play cricket, 20 play foot ball and hockey, 25 play foot ball and cricket, 15 play hockey and cricket and 8 play all the three games. Find the total number of students in the group (Assume that each student in the group plays at least one game).
Let F, H and C represent the set of students who play foot ball, hockey and cricket respectively.
n(F) = 65, n(H) = 45, n(C) = 42,
n(FnH) = 20, n(FnC) = 25, n(HnC) = 15
n(FnHnC) = 8
Total number of students in the group is n(FuHuC).
n(FuHuC) is equal to
= n(F) + n(H) + n(C) - n(FnH) - n(FnC) - n(HnC) + n(FnHnC)
n(FuHuC) = 65 + 45 + 42 -20 - 25 - 15 + 8
n(FuHuC) = 100
Hence, the total number of students in the group is 100.
Alternative Method (Using Venn diagram) :
Venn diagram related to the information given in the question :
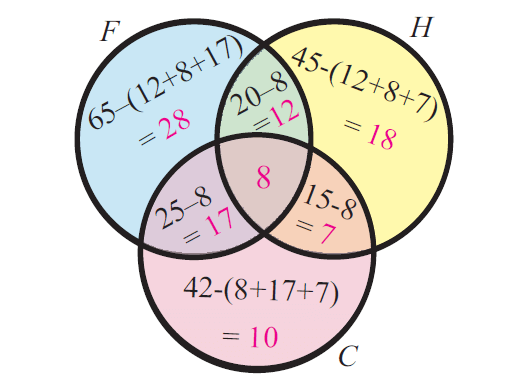
Total number of students in the group :
= 28 + 12 + 18 + 7 + 10 + 17 + 8
So, the total number of students in the group is 100.
Problem 3 :
In a college, 60 students enrolled in chemistry,40 in physics, 30 in biology, 15 in chemistry and physics,10 in physics and biology, 5 in biology and chemistry. No one enrolled in all the three. Find how many are enrolled in at least one of the subjects.
Let C, P and B represents the subjects Chemistry, Physics and Biology respectively.
Number of students enrolled in Chemistry :
Number of students enrolled in Physics :
Number of students enrolled in Biology :
Number of students enrolled in Chemistry and Physics :
n(CnP) = 15
Number of students enrolled in Physics and Biology :
n(PnB) = 10
Number of students enrolled in Biology and Chemistry :
No one enrolled in all the three. So, we have
n(CnPnB) = 0
The above information can be put in a Venn diagram as shown below.
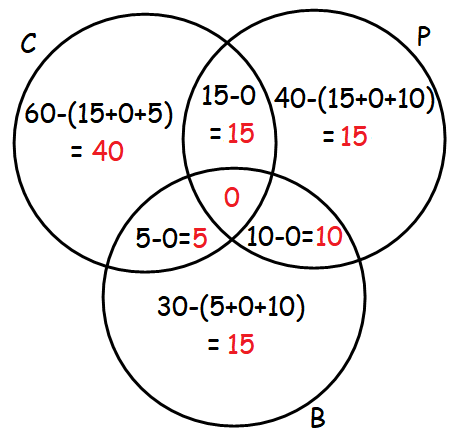
From, the above Venn diagram, number of students enrolled in at least one of the subjects :
= 40 + 15 + 15 + 15 + 5 + 10 + 0
So, the number of students enrolled in at least one of the subjects is 100.
Problem 4 :
In a town 85% of the people speak Tamil, 40% speak English and 20% speak Hindi. Also 32% speak Tamil and English, 13% speak Tamil and Hindi and 10% speak English and Hindi, find the percentage of people who can speak all the three languages.
Let T, E and H represent the people who speak Tamil, English and Hindi respectively.
Percentage of people who speak Tamil :
Percentage of people who speak English :
Percentage of people who speak Hindi :
n(H) = 20
Percentage of people who speak English and Tamil :
n(TnE) = 32
Percentage of people who speak Tamil and Hindi :
n(TnH) = 13
Percentage of people who speak English and Hindi :
n(EnH) = 10
Let x be the percentage of people who speak all the three language.
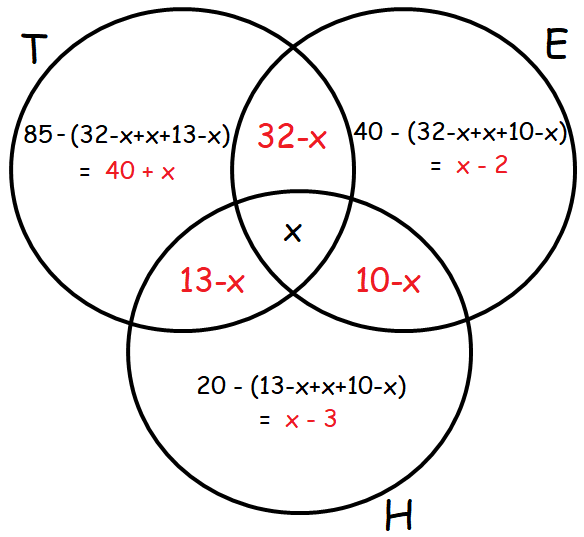
From the above Venn diagram, we can have
100 = 40 + x + 32 – x + x + 13 – x + 10 – x – 2 + x – 3 + x
100 = 40 + 32 + 13 + 10 – 2 – 3 + x
100 = 95 – 5 + x
100 = 90 + x
x = 100 - 90
x = 10%
So, the percentage of people who speak all the three languages is 10%.
Problem 5 :
An advertising agency finds that, of its 170 clients, 115 use Television, 110 use Radio and 130 use Magazines. Also 85 use Television and Magazines, 75 use Television and Radio, 95 use Radio and Magazines, 70 use all the three. Draw Venn diagram to represent these data. Find
(i) how many use only Radio?
(ii) how many use only Television?
(iii) how many use Television and Magazine but not radio?
Let T, R and M represent the people who use Television, Radio and Magazines respectively.
Number of people who use Television :
Number of people who use Radio :
Number of people who use Magazine :
Number of people who use Television and Magazines
n (TnM) = 85
Number of people who use Television and Radio :
n(TnR) = 75
Number of people who use Radio and Magazine :
n(RnM) = 95
Number of people who use all the three :
n(TnRnM) = 70
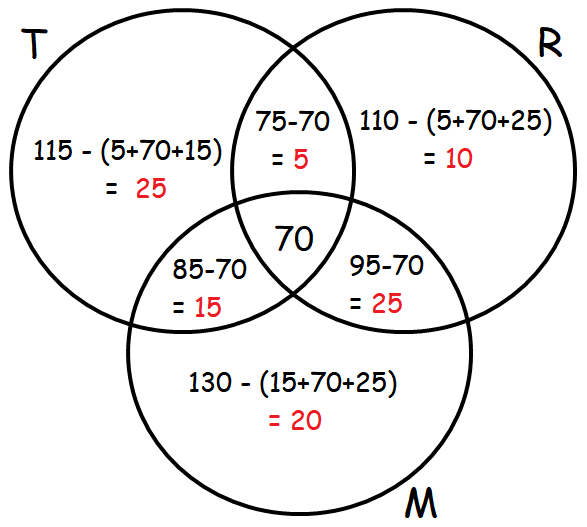
From the above Venn diagram, we have
(i) Number of people who use only Radio is 10.
(ii) Number of people who use only Television is 25.
(iii) Number of people who use Television and Magazine but not radio is 15.
Problem 6 :
In a class of 60 students, 40 students like math, 36 like science, 24 like both the subjects. Find the number of students who like
(i) Math only, (ii) Science only (iii) Either Math or Science (iv) Neither Math nor science.
Let M and S represent the set of students who like math and science respectively.
From the information given in the question, we have
n(M) = 40, n(S) = 36, n(MnS) = 24
Answer (i) :
Number of students who like math only :
= n(M) - n(MnS)
Answer (ii) :
Number of students who like science only :
= n(S) - n(MnS)
= 12
Answer (iii) :
Number of students who like either math or science :
= n(M or S)
= n(MuS)
= n(M) + n(S) - n(MnS)
= 40 + 36 - 24
Answer (iv) :
Total n umber students who like Math or Science subjects :
n(MuS) = 52
Number of students who like neither math nor science
Problem 7 :
At a certain conference of 100 people there are 29 Indian women and 23 Indian men. Out of these Indian people 4 are doctors and 24 are either men or doctors. There are no foreign doctors. Find the number of women doctors attending the conference.
Let M and D represent the set of Indian men and Doctors respectively.
n(M) = 23, n(D) = 4, n(MuD) = 24
n(MuD) = n(M) + n(D) - n(MnD)
24 = 23 + 4 - n(MnD)
n(MnD) = 3
n(Indian Men and Doctors) = 3
So, out of the 4 Indian doctors, there are 3 men.
And the remaining 1 is Indian women doctor.
So, the number women doctors attending the conference is 1.
Kindly mail your feedback to [email protected]
We always appreciate your feedback.
© All rights reserved. onlinemath4all.com
- Sat Math Practice
- SAT Math Worksheets
- PEMDAS Rule
- BODMAS rule
- GEMDAS Order of Operations
- Math Calculators
- Transformations of Functions
- Order of rotational symmetry
- Lines of symmetry
- Compound Angles
- Quantitative Aptitude Tricks
- Trigonometric ratio table
- Word Problems
- Times Table Shortcuts
- 10th CBSE solution
- PSAT Math Preparation
- Privacy Policy
- Laws of Exponents
Recent Articles
SAT Math Resources (Videos, Concepts, Worksheets and More)
Nov 21, 24 06:23 AM
Digital SAT Math Problems and Solutions (Part - 75)
Nov 21, 24 06:13 AM
Digital SAT Math Problems and Solutions (Part - 74)
Nov 20, 24 08:12 AM

IMAGES
VIDEO